
ABSTRACT
There is an increasing awareness to protect historical monuments, in future earthquakes. Taj Mahal is one such monument known for its cultural heritage worldwide and must be protected from damage. The strength evaluation of the structure taking into account future earthquake forces besides gravity and other loads is of concern for determining its safety. The seismic evaluation forms the essential step for retrofitting of such structures under occasional earthquake loads. In the present study, dynamic analysis of the Taj Mahal monument has been carried out on the basis of two simplified 3D mathematical models for fixed and flexible base condition using IS code and site dependent spectra. The free vibration characteristics and seismic response for bending moments, bending stresses, torsional moments, shear forces and axial forces have been studied.
KEYWORDS
Taj Mahal; monument; 3D mathematical model, setsmtc analysis; response spectra; seismic response; safety evaluation
INTRODUCTION
The Taj Mahal in one of the finest architectural monuments of the world and has been included in the cultural treasures of the world heritage. Because of the increasing awareness to protect the historical monuments and its importance, seismic safety evaluation of the marble mausoleum is of great value for its future safety against possible earthquakes.
THE TAJ MAHAL MONUMENT
The monument lies in the seismic zone lii of India on the bank of river Yamuna in the historical city of Agra. The structure has survived small to moderate earthquakes in its life. The Taj Mahal monument (Figure 1) consists of a central main dome resting on two storey thick brick masonry walls square in plan, four small domes each at corner with four 40m high independent minars at the four corners. The whole monument is resting on large platform of thick brick masonry raft which is approximately ISm above the ground level. The center to center distance of columns is 32m and the storey height is measured as 11 m. The foundation soil consists of a deep alluvial deposit.
 |
| Figure 1: Sectional Elevation of Taj Mahal |
MODELING OF THE STRUCTURE
Dynamic analysis of the monument has been carried out for two simplified 3D mathematical models (i) fixed base and (ii) flexible base conditions. The first model is considered fixed at the center of raft while other is considered to be resting on soil springs. Figure 2 shows the 3D mathematical model of the complete structure, each portion of structure wall/ dome are represented by assemblage of beam elements with 6 degree offreedom at each node.
MODELING OF THE FOUNDATION
Type of Soils
There is sandy soil strata beneath the monument having the weight densities 1.8 ton/m3 and 1.6 ton/m3 at masjid site and mehman-khana site respectively. The Poisson's ratio is 0.25. The sandy soil is followed by clayey soil. Both the sandy and clayey soil strata beneath the monument are submerged. No material change in the mechanical properties of the various soil layers would therefore occur due to further impoundment of water in the river Yamuna.
Type of Foundation
The raft foundation is solid square platform measuring approximately l00m x l00m in plan and 35m thick The portion of the raft above the ground level is about 18m. The raft is built in brick masonry consisting of thick fire burnt clay bricks and mortar joints of varying thickness. The platform rests on thick sandy layer followed by clayey layer underneath.In the two mathematical models the bottom most vertical members are assumed to be rigid and half the weight of the raft is lumped at the four bottom nodes
Soil Springs
Based on the meager data published in the literature, soil spring constants for an embedded rnassless rigid rectangular foundation which forms a prism have been used. The increase in stiffness for embedment leads to a factor with which the value for surface foundation is multiplied and which is for surface foundation equal to one. For three different values of shear wave velocities, different soil spring constants are calculated and one fourth of each spring constant is used at all the four bottom most nodes as shown in Fig. 2.
 |
| Fig. 2: Mathematical model of the monument with springs at the bottom nodes |
MATERIAL PROPERTIES
Materials used in the Taj Mahal are sand stone, brick work in lime mortar and marble for cladding. Modulus of elasticity, Poisson's ratio and unit weight densities of all the materials are given in Table 1. The unit weights of soils at masjid site is 1.8 tonfm3 and at mehman-khana site is 1.6 tonfm3. The unit weight of soil for analysis purposes is taken as 1. 7 tonfm3, the average of weight densities at the above two sites. The Poisson's ratio for the soil is taken as 0.25. The analysis has been carried out for three different shear wave velocities, 158 m/sec, 300 rnlsec and 600 m/sec and shear modulii have been calculated accordingly.
 |
| Table 1: Material Properties of Taj Mahal Monument |
The site dependent spectra has been worked out (EQ 92-1 0) at the site of Taj Mahal monument, taking into consideration local soil conditions, geology and seismic history. The zero period acceleration for this spectra is 0.2g while it is 0.1g for code response spectra. The response spectrum analysis has been carried out for both fixed base and flexible base models using IS code spectra and site dependent spectra. For fixed base condition damping of the structure has been taken to be 7% of critical and for flexible base condition 10%. As the city of Agra falls in seismic zone Ill, zone factor is taken as 0.2, importance factor as 1.5 and soil foundation factor as 1.2 for the analysis using code spectra. The digitized values of spectral acceleration (Sa/g) for IS code as well as site dependent spectra are given in Table 2 both for 7% and 10% damping.
 |
| Table 2: Digitized values of spectral acceleration coefficients (Sa/g) for IS code and site dependent spectra |
RESULTS OF ANALYSIS
 |
| Table 3: Time periods of the complete structure for fixed and flexible base conditions |
Dynamic Displacements
The dynamic displacement& of the structure at critical points namely top and bottom of main dome and at the top of small dome for fixed and spring base conditions are given in Table 4 and 5 using code spectra and site dependent spectra respectively. The maximum displacement is at the top of small dome and is equal to 0.87 cm and 8.44 cm for code and site dependent spectra respectively. While at the top of main dome the displacement is 0.29 cm and 2. 77 cm for code and site dependent spectra respectively. The displacement at top of small dome is more than the top of main dome which is due to the fact that the main dome is more rigid than small dome.
 |
| Table 4. Displacement& at critical locations in X-direction using code spectra |
 |
| Table 5. Displacements at critical locations in X-direction using site dependent spectra |
Bending Stresses
The bending stresses at the bottom of main and small domes for fixed and spring base conditions are given in Table 6 and 7 using code spectra and site dependent spectra respectively. It is observed that stresses developed are more in fixed base model as compared to the three cases of the spring base model.
 |
| Table 6. Bending stresses at critical sections using code spectra |
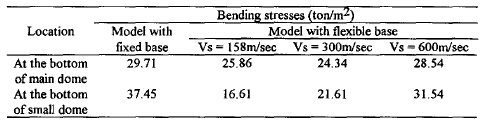 |
| Table 7. Bending stresses at critical sections using site dependent spectra |
CONCLUSIONS
