‘Least squares’ is a powerful statistical technique that may be used for ‘adjusting’ or estimating the coordinates in survey control networks. The term adjustment is one in popular usage but it does not have any proper statistical meaning. A better term is ‘least squares estimation’ since nothing, especially observations, are actually adjusted. Rather, coordinates are estimated from the evidence provided by the observations.
The great advantage of least squares over all the methods of estimation, such as traverse adjustments, is that least squares is mathematically and statistically justifiable and, as such, is a fully rigorous method.
It can be applied to any over determined network, but has the further advantage that it can be used on one-, two- and three-dimensional networks.A by-product of the least squares solution is a set of statistical statements about the quality of the solution. These statistical statements may take the form of standard errors of the computed coordinates, error ellipses or ellipsoids describing the uncertainty of a position in two or three dimensions, standard errors of observations derived from the computed coordinates and other meaningful statistics described later.
The major practical drawback with least squares is that unless the network has only a small number of unknown points, or has very few redundant observations, the amount of arithmetic manipulation makes the method impractical without the aid of a computer and appropriate software.
The examples and exercises in this material use very small networks in order to minimize the computational effort for the reader, while demonstrating the principles. Real survey networks are usually very much larger.
A‘residual’ may be thought of as the difference between a computed and an observed value. For example, if in the observation and estimation of a network, a particular angle was observed to be 30°0' 0'' and after adjustment of the network the same angle computed from the adjusted coordinates was 30° 0' 20'', then the residual associated with that observation would be 20''. In other words:
computed value − observed value = residual
Any estimation of an over determined network is going to involve some change to the observations to make them fit the adjusted coordinates of the control points. The best estimation technique is the one where the observations are in best agreement with the coordinates computed from them. In least squares, at its simplest, the best agreement is achieved by minimizing the sum of the squares of the weighted residuals of all the observations.
2. Least squares applied to engineering
In practical survey networks, it is usual to observe more than the strict minimum number of observations required to solve for the coordinates of the unknown points. The extra observations are ‘redundant’ and can be used to provide an ‘independent check’ but all the observations can be incorporated into the solution of the network if the solution is by least squares.
All observations have errors so any practical set of observations will not perfectly fit any chosen set of coordinates for the unknown points.
Some observations will be of a better quality than others. For example, an angle observed with a 1'' theodolite should be more precise than one observed with a 20'' instrument. The weight applied to an observation, and hence to its residual, is a function of the previously assessed quality of the observation.
In the above example the angle observed with a 1" theodolite would have a much greater weight than one observed with a 20" theodolite. How weights are calculated and used will be described later.
If all the observations are to be used, then they will have to be ‘adjusted’ so that they fit with the computed network. The principle of least squares applied to surveying is that the sum of the squares of the weighted residuals must be a minimum.
2.1 A simple illustration
A locus line is the line that a point may lie on and may be defined by a single observation. Figure1(a), (b) and (c) show the locus lines associated with an angle observed at a known point to an unknown point, a distance measured between a known point and an unknown point and an angle observed at an unknown point between two known points respectively. In each case the locus line is the dotted line. In each case all that can be concluded from the individual observation is that the unknown point lies somewhere on the dotted line, but not where it lies.
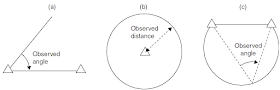 |
| Figure 1 Locus lines |
 | |
|
In the following, the coordinates of new point P are to be determined from horizontal angles observed at known points A, B, C and D as in Figure 2(a). Each observation may be thought of as defining a locus line. For example, if only the horizontal angle at A had been observed then all that could be said about P would be that it lies somewhere on the locus line from A towards P and there could be no solution for the coordinates of P. With the horizontal angles at A and B there are two locus lines, from A towards P and from B towards P. The two lines cross at a unique point and if the observations had been perfect then the unique point would be exactly at P. But since observations are never perfect when the horizontal angles observed at C and D are added to the solution the four locus lines do not all cross at the same point and the mismatch gives a measure of the overall quality of the observations. Figure 2(b) shows the detail at point P where the four lines intersect at six different points. The cross is at the unique point where the sum of the squares of the residuals is a minimum.
2.2 The mathematical tools
By far the easiest way to handle the enormous amounts of data associated with least squares estimation is to use matrix algebra. In least squares it is necessary to create a system of equations with one equation for each observation and each ‘observation equation’ contains terms for each of the coordinates of each of the unknown points connected by the observation. So, for example, in a two-dimensional network of 10 points where there are a total of 50 observations there would be a set of 50 simultaneous equations in 20 unknowns. Although this represents only a small network, the mathematical problem it presents would be too difficult to solve by simple algebraic or arithmetic methods.
